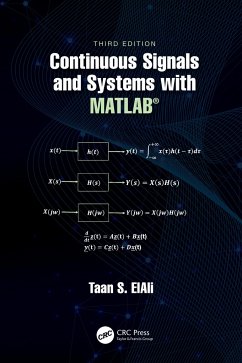
Continuous Function
Versandkostenfrei!
Versandfertig in 6-10 Tagen
26,99 €
inkl. MwSt.
PAYBACK Punkte
13 °P sammeln!
In mathematics, a continuous function is a function for which, intuitively, small changes in the input result in small changes in the output. Otherwise, a function is said to be discontinuous. A continuous function with a continuous inverse function is called bicontinuous. An intuitive though imprecise (and inexact) idea of continuity is given by the common statement that a continuous function is a function whose graph can be drawn without lifting the chalk from the blackboard. Continuity of functions is one of the core concepts of topology, which is treated in full generality in a more advanc...
In mathematics, a continuous function is a function for which, intuitively, small changes in the input result in small changes in the output. Otherwise, a function is said to be discontinuous. A continuous function with a continuous inverse function is called bicontinuous. An intuitive though imprecise (and inexact) idea of continuity is given by the common statement that a continuous function is a function whose graph can be drawn without lifting the chalk from the blackboard. Continuity of functions is one of the core concepts of topology, which is treated in full generality in a more advanced article. This introductory article focuses mainly on the special case where the inputs and outputs of functions are real numbers. In addition, this article discusses the definition for the more general case of functions between two metric spaces. In order theory, especially in domain theory, one considers a notion of continuity known as Scott continuity. Other forms of continuity do existbut they are not discussed in this article. As an example, consider the function h(t) which describes the height of a growing flower at time t. This function is continuous.
Andere Kunden interessierten sich für
